A Simple Game: Hawks and Doves

Synopsis: Here you will have a chance to apply what you havelearned about games and their solution to a classic two strategy game --Hawks and Doves. You will be introduced to these strategies which have utilityin understanding how fighting and display strategies could co-exist in apopulation. After this introduction, you will be guided through the constructionof a payoff matrix which you will use to determine whether or not Hawk orDove are pure ESSs. You will also be introduced to a graphical depictionof evolutionary games. This page marks the end of your "basictraining" in game theory and is the gateway to using the simulationsprovided at this website.

Contents:

• Introduction
• Description of the Hawk and Dove Strategies
• A Qualitative Solution to the Hawk vs. Dove Game
• Construction of the Hawk vs. Dove Game
• Listing of Benefits and Costs
• Calculation of the Fitness of Each Strategy
• Graphical Results of a Simple Game
• Direct Mathematical Solution of an Equilibrium
• An Overview and Gateway to the Hawks Doves Java Simulation
• Bottom of the Page -- Links to other pages, end notes, and copyright notice

Introduction

In the last section, we learned the basics of setting up and solvinga two strategy game. However, we did not actually construct and solve agame.

In this section, we will construct a classic but very simple game knownas Hawks and Doves. These two simplified behavioral strategies employvery different means to obtain resources -- fighting in Hawks and displayin Dove. These differences in behavior have marked consequences on the chanceof winning and of paying certain types of costs. This leads to very differentpayoffs.

Goals and How to Use This Frame: Use the Hawks and Dovesexample to solidify your understanding of basic game theory. As you go throughthis page, links will be provided to get you quickly to various review topics.Your fundamental goal should be to feel thoroughly comfortable with thebasic concepts of evolutionary game theory and with solutions to two strategygames. Gaining this understanding will allow you to get far more outthe simulations available at this website.

In addition, as you study the material on this frame:

• Think hard about what species may use strategies like Dove, Hawk or a mix. Try to move beyond the game to application to real animals.
• One of the most important things you should do is to think about how the relative values of the factors that determine the payoffs ultimately affect the equilibrial point of the Hawks and Doves game. An important part of your use of the simulations will be to check out your ideas about how setting different benefits and costs will change the equilibrial point of a two strategy game.
• Think about the limitations of simple models like Hawks and Doves, yet at the same time be sure to think about the insights it has given you.

Return to this frame's contents and top

Game Theory and EvolutionaryStable Strategies -- An Example Using Alternative Strategies in a SymmetricalContest

As with any game model, our central question is whether or not DOVEand HAWK can coexist and if so, at what frequencies.

Here is a description of the two alternative behaviors:

HAWK: very aggressive, always fights for some resource.

• Fights between hawks are brutal affairs with the loser being the one who first sustains injury. The winner takes sole possession of the resource.
• Although hawks that lose a contest are injured, the mathematics of the game requires that they not die and in fact are fully mended before their next expected contest (see note)
• For simplicity, we will assume that all hawks are equal in fighting ability; that is, each hawk has a 50% chance of winning a Hawk - Hawk conflict. Another way of saying this is that Hawk vs. Hawk contests are symmetrical.

DOVE: never fights for a resource -- it displays inany conflict and if it is attacked it immediately withdraws before itgets injured.

• Thus, in any conflict situation, dove will always lose the resource to a hawk, but it never gets hurt (never sustains a decrease in fitness) when confronting a hawk, and therefore the interactions are neutral with respect to the dove's fitness (see note).
• A corollary to this rule is that doves do not display for very long against hawks. After starting their displays, they immediately recognize that their opponent is a hawk and they withdraw without paying a meaningful display cost. (press here to review the concept of display costs)
• On the other hand, if a dove meets a dove there will be a period of displaying with some cost (time, energy for display) but no injury. We assume that all doves are equally good at displaying and they adapt a strategy of waiting for a random time period (see "War of Attrition Game"). Therefore when two doves face off, each has a 50% chance of winning.
• Notice that both doves will pay essentially the same display cost in any contest. The winner is the individual willing to pay more. However, note that the winner quits displaying essentially at the same time as the loser withdraws (see war of attrition game)

! Notice that we have assumed there are no asymmetries within a strategy -- all hawks are equallygood at fighting and all doves are equally good at displays. An animal thatwins one contest is just as likely to win or to lose the next. Thus in anycontest between members of the same strategy, either contestant has an equalchance of winning -- there is no correlation with past success, condition,whatever. This is clearly not a very reasonable assumption, but we're juststarting out so let's keep things simple.

TWO OTHER IMPORTANT ASSUMPTIONS:

• Assume that the attacking animal (the one that either starts first to physically attack or to display) has no knowledge of the strategy that its opponent will play.
• Assume that these interactions increase or decrease the animal's fitness from some baseline fitness. In other words, these interactions simply modify up or down an animal's fitness -- wining a contest does create fitness. This assumption is associated with our convention that injuries and display costs will be assigned negative scores -- losing animals do not have negative fitnesses! (to review discussion of this idea with regards to modeling in general, press here).

Return to this frame's contents and top

Preliminary (Qualitative) Exploration

First, we'll make a qualitative analysis of the game, then we'll usegame theory to make a much more quantitative prediction (as was discussedin the introductorymaterial dealing with games) . Let's start with the following question:Are either of the two strategies by themselves impervious to invasion? --that is, does either represent a pureESS?

To most people it immediately appears that DOVE is not a pure ESS.Imagine a population entirely of doves. It is probably a very nice placeto live and everyone is doing reasonably well without injuries when it comesto conflicts over resources - the worst thing that happens to you is thatyou waste time and energy displaying. But that is OK, because on the averageyou win 50% of the encounters. Therefore on the average, you will come outahead provided the display costs are not large compared to the resourcevalue.

Now, imagine what happens if a HAWK appears by mutation or immigration.The Hawk will do extremely well relative to any dove -- winning every encounterand initially at least suffering no injuries. Thus, its frequency will increaseat the expense of dove. Thus, Dove is not a pure ESS. If dove isnot an ESS, what about hawk?

So, let's do the analysis again, this time starting with a populationmade entirely of hawks. This would be a nasty place, an asphalt junglewhere you would not want to live. Lots of injurious fights. Although thesefights don't kill you, they tend to lower everyone's fitness. Yet, justlike with the dove population, no hawk is doing better than any other andthe resources are getting divided equally.

Could a DOVE possibly invade this rough place? It might not seem so sincethey always lose fights with hawks. Yet think about it:

• Doves don't get hurt -- the best way to think about this is that they do not pay high costs in fitness for losing a contest. While they never beat a hawk, they don't get hurt because they flee the moment they realize they are in a contest with a hawk. The result is that, unlike hawk-hawk contests, a dove's fitness is not lowered by a conflict with a hawk (i.e., it is the same as if no contest at all had occurred -- remember that we assume a certain baseline of fitness independent of the outcome of contests - contests only increase or decrease this amount).
• Doves are winners 50% of the time against other doves and they probably lose little in such contests.

Thus, if a mutant appears in the form of a dove or one wanders in fromelsewhere, it will do quite well relative to hawk and increase in frequency.Thus, Hawk is also not a pure ESS.

Notice that in all of the arguments above, we made implicit assumptionsabout the relative values of the resource and the costs of injury and displaythat are consistent with the behavioral descriptions . You probably realizethat if we changed some of these assumptions of relative value, the gamemight turn out differently -- perhaps Hawk or Dove could become an ESS.Moreover, even if we stick to the qualitative values and to our conclusionthat there is no pure ESS, the technique we have just used will not allowus to predict the frequencies of Dove and Hawk at the mixed ESS. As wasstated earlier, the best models make quantitative predictions since theseare often most easily tested (to review testing of models, press here).

Thus, in the next section we will use the rules and techniques we previouslylearned to quantitatively analyze the Hawks and Doves game.

Return to this frame's contents and top

Formal Analysis of the Hawk-DoveESS

The first step of our analysis is to set-up a payoff matrix. Recall that the matrix lists the payoffsto both strategies in all possible contests:

	Opponent
Focal Strategy	Hawk	Dove
Hawk	E(H,H)	E(H,D)
Dove	E(D,H)	E(D,D)

We now need to make explicit how we arrive at each payoff. Recall thatthe general form of an equation used to calculate payoffs (press here to review) is:

Eq. 1. Payoff(to Strat., when vs. aStrat.) = [(chance of win) * (resource value - cost of win)] + [(chance of loss) * cost of loss]

We will use thedescriptions of the strategies given previously to write the equationsfor each payoff. But first, let's assign some benefits and costs (we coulddo this later, but let's do it now so that we can calculate each payoffas soon as we write its equation):

Action	Benefit or Cost (arbitrary units)
Gain Resource	+ 50
Lose Resource	0
Injury to Self	- 100
Cost of Display to Self	- 10

Rationale for these values:

Gain resource -- self-explanatory.

Lose resource -- self-explanatory (nothing gained).

Injury to self -- if the injury cuts into the animal's abilityto gain the resource in the future, then the cost of an injury is assesedas a large negative. That is, injury now tends to preclude gain in the future.On the other hand, if there is one and only one chance to gain the resource,should severe injury or death be given a large negative value? Think aboutthis, we'll revisit this situtation when we run the Hawk and Dove simulation.

? In the list of cost and benefits above, it is assumed thatinjury costs are large compared to the payoff for gaining the resource.Give a situation where this relative weighing might accurately reflect theforces acting on an animal. ANS

Cost of display -- displays generally have costs, although howhigh they are varies -- clearly they have variable costs in terms of energyand time and they may also increase risk of being preyed upon. All of thesetype of measurements, in theory at least, can be translated into fitnessterms.

! Important Note: All of these separate payoffs are in unitsof fitness (whatever they are!). You will see shortly that the values thatare assigned to each payoff is crucial to outcome of the game -- thus accurateestimates are vital in usefulness of any ESS game in understanding a behavior.

a. Calculation of the payoff to Hawk in Hawk vs. H contests: Relevant variables (from eq. 1)

• chance of winning (50% -- i.e. the contests are symmetrical)
• resource value (see table)
• chance of losing (50%)
• costs of losing -- in this case, the cost is an injury cost (see table)
• notice that no costs are paid in winning

? Does it seem reasonable that hawks pay no cost in winning?Also, does it seem reasonable that the loser only pays an injury cost? Thinkabout what animals do and about simplifications of models. Forsome discussion of this question, press here (but think about it first)

Thus:

Eq. 2: E(H,H) = (0.5 * 50) + (0.5 * -100) = 25 - 50 = -25

Note; the costs of losing are added in our model sincewe gave the costs anegative sign to emphasize that they lowered the fitness of theloser.

b. Calculation of the payoff to Hawk when vs. Dove:
Relevant variables (from eq. 1)

• chance of winning 100% -- i.e. the contests are asymmetrical
• resource value (see table)
• no costs to the hawk since (a) they never lose and (b) since the dove immediately retreats once it recognizes the hawk

Eq. 3: E(H,D) = 1.0 * 50 - 0 = +50

c. Calculation of the payoff to Dove when vs. Hawk:
Relevant variables (from eq. 1)

• chance of winning o% -- i.e. the contests are asymmetrical and doves always lose
• no costs to the dove since it immediately retreats once it recognizes the hawk

Eq. 4: E(D,H) = 0 * 50 + 1.0 * 0 = 0

d. Calculation of the payoff to Dove when vs. Dove:
Relevant variables (from eq. 1)

• chance of winning (50% -- dove vs. dove contests are symmetrical)
• resource value (see table)
• display cost paid in winning -- both animals will display essentially the same amount of time. The one that wins is the one that is willing to display for a longer period of time at this particular time (see war of attrition).
• chance of losing (50%)
• costs of losing -- as with the winner, the loser also pays a display cost and it is the same as what the winner pays (see table)
• no injury cost -- no violence please, we're doves for heaven's sake!

Eq. 5: E(D,D) = (0.5) * (50 - 10) - (0.5) * (-10) = +15

So for this particular version of the Hawk vs. Dovegame (defined by these payoffs), the pay-off matrix is:

	Opponent
Focal Strategy	Hawk	Dove
Hawk	-25	+50
Dove	0	+15

? Using this matrix, see if the Hawk Dove game above meetsthe criteria for a pure ESS. (hint: reviewthe rules for a pure ESS and then arbitrarily define H as A and testto see if H is a pure ESS with payoffs listed above (Do this for both strategies-- use H and then D as strategy A. Should you get the same results eachtime?) ANS -- as usual,please try to reason through this one before going to the answer.

Return to this frame's contents and top

Calculations of the Fitnessof Each Strategy and Mixed ESSs

If you did the problem above, you will realize that neither Hawk norDove are pure ESSs given the payoffs calculated from the equations and valuesfor benefits and costs presented above. (When you use the simulation, youwill see that certain benefits and costs can be used to make either of thestrategies pure ESSs, although these might seem to involve unreasonableassumptions).

It is good to keep in mind the fact that the rules you used to determinethat neither strategy was a pure ESS require some reasonable assumptions(to review, presshere).

If we have no pure ESS, we know that in a two strategy game there willbe a mixed ESS which is defined as the frequencies of the strategies whereboth have equal fitness. Recall that the fitness of a strategy is the sumof the payoffs times the frequency of their occurrence

Thus, if we assume that:

Eq. 7a: frequency(Hawk) = h   then: Eq. 7b: frequency(Dove) = (1 - h)

Thus, the fitness of Hawk, W(H), is:

Eq. 8: W(H) = h * E(H,H) + (1-h)* E(H,D)

and the fitness of Dove, W(D) is:

Eq. 9: W(D) = h * E(D,H) + (1-h) * E(D,D)

Notice that each of the equations for strategy fitness yield a straightline when solved for a series of frequencies.

Now since IN A MIXED ESS BOTH STRATEGIES MUSTHAVE THE SAME FITNESS, we can determine the equilibrial mix by settingthe fitnesses of the two strategies as equal to each other:

Eq. 10: W(H) = W(D) at equilibrium (mixed ESS)

For our game:

Eq. 11: h * E(H,H) + (1 - h) * E(H,D) = h * E(D,H) + (1 - h) * E(D,D)

If we now solve for the frequency of hawk at this equilibrium:

Eq. 12: h / (1 - h) = [E(D,D) - E(H,D)] / [E(H,H) - E(D,H)]

? Calculate the mixed ESS frequencies of Hawk and Dove using thepayoff matrix above.ANS.

We can understand the solution more clearly if we graph eqs. 8 and 9where the solid line is dove (eq. 9) and the dotted line is hawk(eq. 8). The intersection of the Hawk and Dove plotsrepresents the frequency of one strategy (in this case Hawk) where the fitnessesof both strategies are equally fit (in terms of payoff units).

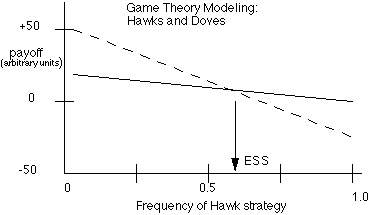
Remarks About the Graphical Results
ofthe Hawk vs. Dove Game:

The above graph points up a number of interesting things:

• Note that at the equilibrial point, the addition of individuals of either strategy lowers the relative fitness of all members of that strategy.
  • Thus, an increase in Hawks results in moving to the right along the hawk line to a lower fitness than that of dove -- away from equilibrium.
  • Likewise, addition of Doves (movement to the left along the Dove line since their frequency is (1 - h) lowers their fitness relative to Hawk.
  • In both cases, the lowered fitness will eventually result in a reduction of their numbers and a return to the equilibrium frequency.
• Also note that the addition of any Hawk overall lowers everyone's absolute fitness! -- both curves have negative slopes.
• And, the graph provides another way to see that neither Hawk nor Dove are pure ESSs:
  • Hawk does very poorly when in high frequency compared to when it is rare. Thus, it is easily invaded by what might seem to be the most improbable of invaders, the pacific "dove".
  • On the other hand, Dove is not stable since Hawks do extremely well when entering the population
  • Thus, although it is obvious that any Hawks would always be "bad for the species" they cannot be kept out once they appear (so much for group selection being a common phenomenon). For this particular set of payoffs, Dove is not an ESS any more than Hawk is.

Return to this frame's contents and top

Computer Simulation of the Hawksand Dove Game

At this point you know how to set up and solve a simple game. And youhave a basic familiarity with the Hawks and Doves game.

So, you are now ready to explore the Hawks and Doves game in detail usinga simulation that will allow you to alter payoffs by changing benefits andcosts. The simulation will provide you with a visual representation of thesolution, using the same techniques you have just learned (except the computerwill now do the computational work for you!). And, you'll get to see somethingnew -- you'll be able to set frequencies of the two strategies and thensee how a population with a given payoff matrix will evolve over time.

Press here to go to apage that explains how to use the simulation and then launches it.

End Notes

Why can't hawks die or get permanentlyknocked out of action? Why must they be miraculously restoredto health?

The reason is very simple. If this were not the case, then in any populationcontaining more than one hawk, Hawk vs. Hawk contests would cause the frequencyof hawk to decrease. The more hawks, the more Hawk vs. Hawk contests andthe faster freq(Hawk) will decrease! Notice that the equations we learnedearlier for finding the fitness of the strategy all implied a constant freq.of the strategy. Thus, the bad things that happen in Hawk vs. Hawk contestsshould be seen as changing (in this case lowering -- to see one exampleof a Hawk vs. Dove payoff matrix press here) the general fitness of hawk individuals in thepopulation without changing their frequencies.

Return to previous place in MAIN text

Return to previous place in end notes

There are a couple of things to notice here. First,no doves get killed. To reiterate the material about freq of Hawk and injury,notice that if injured hawks did drop out, the freq. of Dove would increase.Also notice the difference in the payoff (according to the descriptionsof H and D that you have just read or in the same example payoff matrix that we considered with Hawk)-- negative payoffs tend to mean a lowered fitness as a result of the contestbut not death; and payoffs of 0 (the payoff to Dove vs. Hawk in this example)mean no effect on fitness -- the dove goes on as before.

Return to previous place in text

The important idea here is that the animal mustbe able to reproduce even if it loses all of its contests. If not, you mightas well count the animal as dead with the same consequences as outlinedin the discussion of injuries. Again, the importantconsequence of the game is that it alters the fitness of the individualbut does not kill (or essentially kill) the individual.

Return to previous place in text

Go to:

• Top of the page
• Continue your studies -- a third strategy -- Bourgeois
• Definition Page

Copyright © 1999 by Kenneth N. Prestwich College of the Holy Cross, Worcester, MA USA 01610email: kprestwi@holycross.edu About FairUse of these materials Last modified 12 - 1 - 09